Dust Continuum Observing Strategy

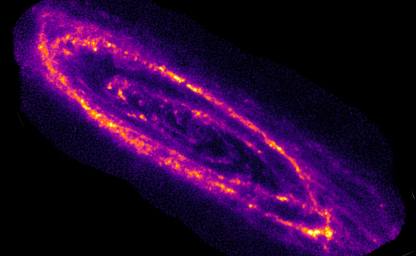
The HASHTAG observing strategy was based on our experience of from our SCUBA-2 pilot survey in 2015. For the pilot field we choose to use a 30 arcmin (diamter) pong as this provided a good balance between depth and including a significant background region. Each observation is observed for 43 minutes, which we then repeated 37 times. This produced mapswith a sensitivity of 44.9 mJy beam-1 and 3.0 mJy beam-1, at 450 and 850µm, respectively (using 2.0 and 4.0 arcsecond pixels). Our initial reduction is shown in the Figure below.

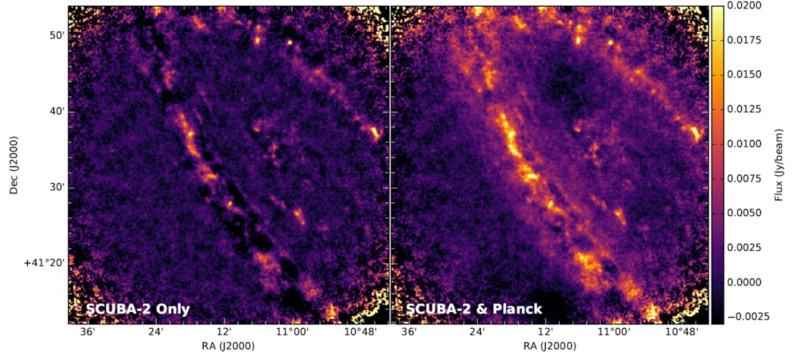
A limitation of ground-based sub-mm astronomy is that due to atmosphere variations filtering is required which attenuates emission on the largest-angular scales. Ground-based observations are needed as we can build much bigger telescopes on the ground than we can put in space (and hence much better resolution). To overcome the limitations of the atmosphere we take the lower-Resolution space-based date (but includes the large-scale emission), and combine it with the ground-based data to obtain an image with both the high-resolution and large-scale information (using a feathering technique). For more information on this process see our data reduction paper, and our software page.

Feathering
An example of our feathering technique applied to the our pilot field which observed a 30ยด region of M31. The left image shows the image made just with SCUBA-2 data, the right image is the same SCUBA-2 data combined with space-based Planck data.

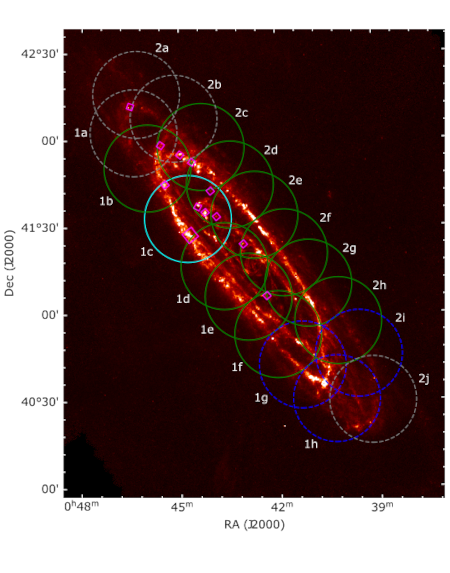
For the full HASHTAG project we decided to keep to a similar strategy, again using 30 arcmin pongs including similar areas of 'background'. To cover the entirity of Andromeda we created a set of 18 overlapping pongs with two rows just above and below the major axis (see Figure below). At all positions every point is covered by at least two pong positions, with each pong repeated 17 times (half our pilot field). This strategy has many advatantages; first it helps ensure we do not have areas of low coverage, second as the two rows overlap in the centre we have good sensitivity in an area where we expect less dust, and third the large number of overlaps between observations is optimal for the data processing. As we are also interested in looking for transients we try to have a temporal gap between sets of repeats.

Survey Strategy
The HASHTAG observing plan superimposed on the Herschel 250µm image (Smith et al. 2012). Each circle represents one of our 300 Pong observations, each identified by a white label. The color of the circle represents the observing status of the Pong when the DR1 products were constructed (November 2020): green shows pongs for which all 17 observations have been completed; dashed blue shows roughly half (eight or nine), have been completed; and dashed grey indicates no observation has yet been made (1a has one observation). The cyan circle (1c) is our 'pilot' field from 2015 which has 37 observations, which is why there is less overlap with the other circles.